
The reflection of light from varnish and paint layer interfaces is sketched in Figure 6.4. Light is reflected when it is incident on a surface or interface between two different materials such as the surface between air and water, or air and paint, or varnish and paint. The smooth varnished surface of the painting shown in cross section in Figure 6.3a causes specular reflection and appears shiny, while the rough surface of the painting in Figure 6.3b has diffuse reflective properties and appears matte, or dull. The surface of paint layers reflects light in much the same way as water. Reflection from such a rough surface is called diffuse reflection. Consequently, the outgoing rays are reflected at many different angles, and the image is disrupted. The law of reflection is obeyed, but the incident rays strike different regions that are inclined at different angles to each other (Figure 6.2b). When the surface of water is wind-blown and irregular, the rays of light are reflected in many directions. We call the reflection from a smooth, mirrorlike surface specular (as shown in Figure 6.2a). Just as images are reflected from the surface of a mirror, light reflected from a smooth water surface also produces a clear image. Relationship that the angle of incidence equals the angle of reflection. The rough surface (diffuse reflection) will appear matte. Cross sections of paintings with (a) varnish-covered surface and (b) rough paint surface without a varnish overlayer. In both cases the angle of incidence equals the angle of reflection at the point that the light ray strikes the surface. Light reflection from (a) smooth surface (specular reflection) and (b) rough surface (diffuse reflection). We show in Figure 6.1 that the angles of incidence i and reflection i' are equal by joining the two angles with an equal sign. The law of reflection requires that the two rays be at identical angles but on opposite sides of the normal, which is an imaginary line (dashed in Figure 6.1) at right angles to the mirror surface at the point where the rays meet. In Figure 6.1 we use a single line to illustrate a light ray reflected from a surface. Reflection involves two rays-an incoming, or incident, ray and an outgoing, or reflected, ray.

The dashed line (normal) is perpendicular to the sample surface.įace is reflected.
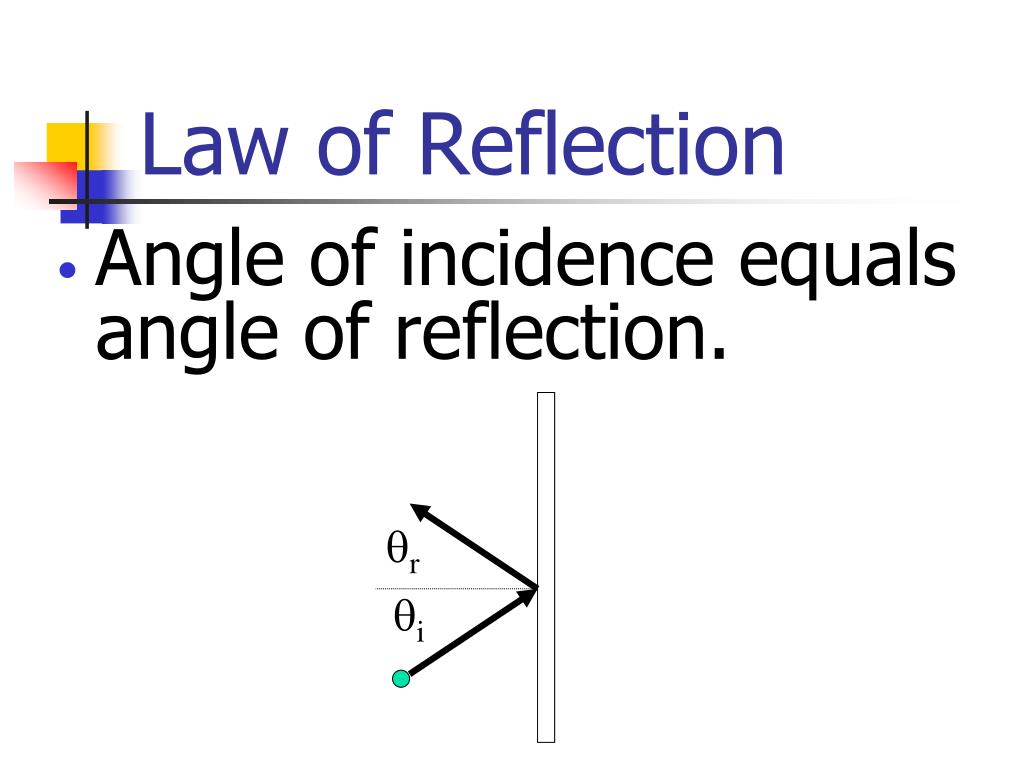
Light ray reflected from a metal surface with angle of incidence i equal to the angle of reflectance i'. A beam of light incident on the metal sur Polished metal surfaces reflect light much like the silver layer on the 66 back side of a glass mirror.


 0 kommentar(er)
0 kommentar(er)
